PriorityQueue 源码解析

1、PriorityQueue概念

• PriorityQueue是优先队列，PriorityQueue实现了Queue接口，不允许元素为null。
• 优先队列的作用是能保证每次取出的元素都是队列中权值最小的，Java默认是取最小的，也可以传入比较器。
• PriorityQueue是通过完全二叉树实现的小顶堆。而堆底层的实现还是数组。

PriorityQueue是有先后优先级的队列。

我们先来回顾一下完全二叉树和堆的概念，也可以去看看相关的一些数据结构和算法。

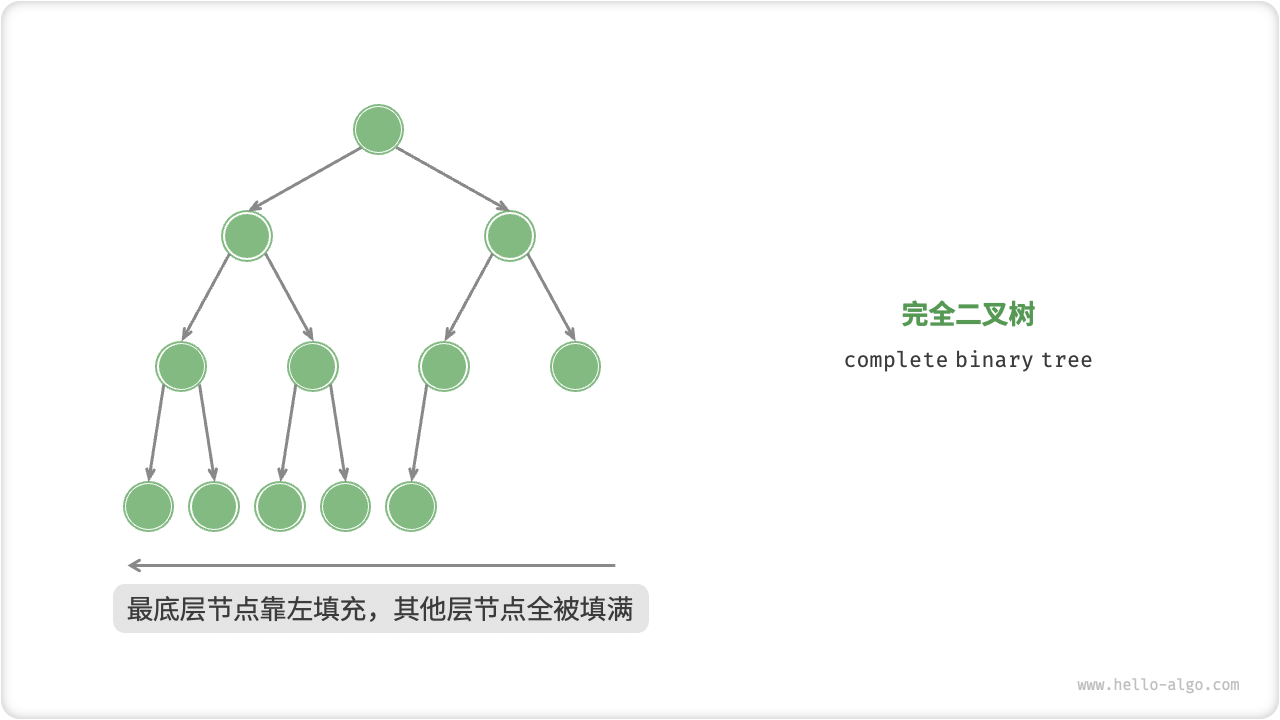
2、完全二叉树

完全二叉树（complete binary tree）是二叉树的其中一种，只有最底层的节点未被填满，且最底层节点尽量靠左填充。

完全二叉树结构

3、堆

堆（heap）是一种满足特定条件的完全二叉树，堆又分为大顶堆、小顶堆。

• 大顶堆：任意节点的值大于等于其子节点的值。
• 小顶堆：任意节点的值小于等于其子节点的值。

堆结构

5、PriorityQueue实现结构

完全二叉树实现的小顶堆，数组实现的优先队列。

通过如下公式计算某个节点的父节点以及子节点的下标

leftNo = parentNo*2+1
rightNo = parentNo*2+2
parentNo = (nodeNo-1)/2

5、PriorityQueue源码解析

PriorityQueue的peek()和element操作是常数时间，add(), offer(), 无参数的remove()以及poll()方法的时间复杂度都是log(N)。

源码

public class PriorityQueue<E> extends AbstractQueue<E>
    implements java.io.Serializable {

    private static final long serialVersionUID = -7720805057305804111L;

    private static final int DEFAULT_INITIAL_CAPACITY = 11;

    /**
     * Priority queue represented as a balanced binary heap: the two
     * children of queue[n] are queue[2*n+1] and queue[2*(n+1)].  The
     * priority queue is ordered by comparator, or by the elements'
     * natural ordering, if comparator is null: For each node n in the
     * heap and each descendant d of n, n <= d.  The element with the
     * lowest value is in queue[0], assuming the queue is nonempty.
     */
    transient Object[] queue; // non-private to simplify nested class access

    /**
     * The number of elements in the priority queue.
     */
    private int size = 0;

    /**
     * The comparator, or null if priority queue uses elements'
     * natural ordering.
     */
    private final Comparator<? super E> comparator;

    /**
     * The number of times this priority queue has been
     * <i>structurally modified</i>.  See AbstractList for gory details.
     */
    transient int modCount = 0; // non-private to simplify nested class access

    // ...
}

可以看到PriorityQueue内部的参数都是比较简单的，只有一个queue（数组存储）、size（元素个数）、comparator（比较器）、modCount（结构修改次数）。

5.1、构造函数

PriorityQueue提供了多个构造函数，可以根据需要选择合适的构造函数。

源码

/**
 * Creates a {@code PriorityQueue} with the default initial
 * capacity (11) that orders its elements according to their
 * {@linkplain Comparable natural ordering}.
 */
public PriorityQueue() {
    this(DEFAULT_INITIAL_CAPACITY, null);
}

/**
 * Creates a {@code PriorityQueue} with the specified initial
 * capacity that orders its elements according to their
 * {@linkplain Comparable natural ordering}.
 *
 * @param initialCapacity the initial capacity for this priority queue
 * @throws IllegalArgumentException if {@code initialCapacity} is less
 *         than 1
 */
public PriorityQueue(int initialCapacity) {
    this(initialCapacity, null);
}

/**
 * Creates a {@code PriorityQueue} with the default initial capacity and
 * whose elements are ordered according to the specified comparator.
 *
 * @param  comparator the comparator that will be used to order this
 *         priority queue.  If {@code null}, the {@linkplain Comparable
 *         natural ordering} of the elements will be used.
 * @since 1.8
 */
public PriorityQueue(Comparator<? super E> comparator) {
    this(DEFAULT_INITIAL_CAPACITY, comparator);
}

/**
 * Creates a {@code PriorityQueue} with the specified initial capacity
 * that orders its elements according to the specified comparator.
 *
 * @param  initialCapacity the initial capacity for this priority queue
 * @param  comparator the comparator that will be used to order this
 *         priority queue.  If {@code null}, the {@linkplain Comparable
 *         natural ordering} of the elements will be used.
 * @throws IllegalArgumentException if {@code initialCapacity} is
 *         less than 1
 */
public PriorityQueue(int initialCapacity,
                        Comparator<? super E> comparator) {
    // Note: This restriction of at least one is not actually needed,
    // but continues for 1.5 compatibility
    if (initialCapacity < 1)
        throw new IllegalArgumentException();
    this.queue = new Object[initialCapacity];
    this.comparator = comparator;
}

/**
 * Creates a {@code PriorityQueue} containing the elements in the
 * specified collection.  If the specified collection is an instance of
 * a {@link SortedSet} or is another {@code PriorityQueue}, this
 * priority queue will be ordered according to the same ordering.
 * Otherwise, this priority queue will be ordered according to the
 * {@linkplain Comparable natural ordering} of its elements.
 *
 * @param  c the collection whose elements are to be placed
 *         into this priority queue
 * @throws ClassCastException if elements of the specified collection
 *         cannot be compared to one another according to the priority
 *         queue's ordering
 * @throws NullPointerException if the specified collection or any
 *         of its elements are null
 */
@SuppressWarnings("unchecked")
public PriorityQueue(Collection<? extends E> c) {
    if (c instanceof SortedSet<?>) {
        SortedSet<? extends E> ss = (SortedSet<? extends E>) c;
        this.comparator = (Comparator<? super E>) ss.comparator();
        initElementsFromCollection(ss);
    }
    else if (c instanceof PriorityQueue<?>) {
        PriorityQueue<? extends E> pq = (PriorityQueue<? extends E>) c;
        this.comparator = (Comparator<? super E>) pq.comparator();
        initFromPriorityQueue(pq);
    }
    else {
        this.comparator = null;
        initFromCollection(c);
    }
}

/**
 * Creates a {@code PriorityQueue} containing the elements in the
 * specified priority queue.  This priority queue will be
 * ordered according to the same ordering as the given priority
 * queue.
 *
 * @param  c the priority queue whose elements are to be placed
 *         into this priority queue
 * @throws ClassCastException if elements of {@code c} cannot be
 *         compared to one another according to {@code c}'s
 *         ordering
 * @throws NullPointerException if the specified priority queue or any
 *         of its elements are null
 */
@SuppressWarnings("unchecked")
public PriorityQueue(PriorityQueue<? extends E> c) {
    this.comparator = (Comparator<? super E>) c.comparator();
    initFromPriorityQueue(c);
}

/**
 * Creates a {@code PriorityQueue} containing the elements in the
 * specified sorted set.   This priority queue will be ordered
 * according to the same ordering as the given sorted set.
 *
 * @param  c the sorted set whose elements are to be placed
 *         into this priority queue
 * @throws ClassCastException if elements of the specified sorted
 *         set cannot be compared to one another according to the
 *         sorted set's ordering
 * @throws NullPointerException if the specified sorted set or any
 *         of its elements are null
 */
@SuppressWarnings("unchecked")
public PriorityQueue(SortedSet<? extends E> c) {
    this.comparator = (Comparator<? super E>) c.comparator();
    initElementsFromCollection(c);
}

可以看到PriorityQueue提供的方法还是比较简单的，有可以传入比较器的，也有可以设置初始化数组大小的。

5.2、add()和offer()

add(E e)和offer(E e)的语义相同，都是向优先队列中插入元素，只是Queue接口规定二者对插入失败时的处理不同，前者在插入失败时抛出异常，后则则会返回false。对于PriorityQueue这两个方法其实没什么差别。

插入过程

新加入的元素可能会破坏小顶堆的性质，因此需要进行必要的调整。

源码

//offer(E e)
public boolean offer(E e) {
    if (e == null)//不允许放入null元素
        throw new NullPointerException();
    modCount++;
    int i = size;
    if (i >= queue.length)
        grow(i + 1);//自动扩容
    size = i + 1;
    if (i == 0)//队列原来为空，这是插入的第一个元素
        queue[0] = e;
    else
        siftUp(i, e);//调整
    return true;
}

上述代码中，扩容函数grow()类似于ArrayList里的grow()函数，就是再申请一个更大的数组，并将原数组的元素复制过去，这里不再赘述。需要注意的是siftUp(int k, E x)方法，该方法用于插入元素x并维持堆的特性。

源码

//siftUp()
private void siftUp(int k, E x) {
    while (k > 0) {
        int parent = (k - 1) >>> 1;//parentNo = (nodeNo-1)/2
        Object e = queue[parent];
        if (comparator.compare(x, (E) e) >= 0)//调用比较器的比较方法
            break;
        queue[k] = e;
        k = parent;
    }
    queue[k] = x;
}

新加入的元素x可能会破坏小顶堆的性质，因此需要进行调整。调整的过程为：从k指定的位置开始，将x逐层与当前点的parent进行比较并交换，直到满足x >= queue[parent]为止。注意这里的比较可以是元素的自然顺序，也可以是依靠比较器的顺序。

5.3、element()和peek()

element()和peek()的语义完全相同，都是获取但不删除队首元素，也就是队列中权值最小的那个元素，二者唯一的区别是当方法失败时前者抛出异常，后者返回null。根据小顶堆的性质，堆顶那个元素就是全局最小的那个；由于堆用数组表示，根据下标关系，0下标处的那个元素既是堆顶元素。所以直接返回数组0下标处的那个元素即可。

获取堆顶元素

源码

//peek()
public E peek() {
    if (size == 0)
        return null;
    return (E) queue[0];//0下标处的那个元素就是最小的那个
}

5.4、remove()和poll()

remove()和poll()方法的语义也完全相同，都是获取并删除队首元素，区别是当方法失败时前者抛出异常，后者返回null。由于删除操作会改变队列的结构，为维护小顶堆的性质，需要进行必要的调整。

删除过程

源码

public E poll() {
    if (size == 0)
        return null;
    int s = --size;
    modCount++;
    E result = (E) queue[0];//0下标处的那个元素就是最小的那个
    E x = (E) queue[s];
    queue[s] = null;
    if (s != 0)
        siftDown(0, x);//调整
    return result;
}

上述代码首先记录0下标处的元素，并用最后一个元素替换0下标位置的元素，之后调用siftDown()方法对堆进行调整，最后返回原来0下标处的那个元素（也就是最小的那个元素）。重点是siftDown(int k, E x)方法，该方法的作用是从k指定的位置开始，将x逐层向下与当前点的左右孩子中较小的那个交换，直到x小于或等于左右孩子中的任何一个为止。

源码

//siftDown()
private void siftDown(int k, E x) {
    int half = size >>> 1;
    while (k < half) {
    	//首先找到左右孩子中较小的那个，记录到c里，并用child记录其下标
        int child = (k << 1) + 1;//leftNo = parentNo*2+1
        Object c = queue[child];
        int right = child + 1;
        if (right < size &&
            comparator.compare((E) c, (E) queue[right]) > 0)
            c = queue[child = right];
        if (comparator.compare(x, (E) c) <= 0)
            break;
        queue[k] = c;//然后用c取代原来的值
        k = child;
    }
    queue[k] = x;
}

5.5、remove(Object o)

remove(Object o)方法用于删除队列中跟o相等的某一个元素（如果有多个相等，只删除一个），该方法不是Queue接口内的方法，而是Collection接口的方法。由于删除操作会改变队列结构，所以要进行调整；又由于删除元素的位置可能是任意的，所以调整过程比其它函数稍加繁琐。具体来说，remove(Object o)可以分为2种情况：1. 删除的是最后一个元素。直接删除即可，不需要调整。2. 删除的不是最后一个元素，从删除点开始以最后一个元素为参照调用一次siftDown()即可。此处不再赘述。

删除过程

源码

//remove(Object o)
public boolean remove(Object o) {
	//通过遍历数组的方式找到第一个满足o.equals(queue[i])元素的下标
    int i = indexOf(o);
    if (i == -1)
        return false;
    int s = --size;
    if (s == i) //情况1
        queue[i] = null;
    else {
        E moved = (E) queue[s];
        queue[s] = null;
        siftDown(i, moved);//情况2
        ......
    }
    return true;
}

参考：深入理解Java PriorityQueue (opens new window)